Constant Rate of
Change 3-1
Quick Review of Proportions
A proportion = 2 equal ratios ( 2 equivalent fractions)
Solve using equivalent fractions or cross multiplication and
then a one-step equation
(see if you can simplify the fractions before you multiply)
Solve the proportion for y
Equivalent Fraction
approach:
Multiply both the top and the bottom by 7
y = 28
Cross Products Approach
you will get
3y = (21)(4)
Now divide each side by 3
DO THIS BEFORE multiplying on the right side? WHY? Because a lot of times you will be able to
cross cancel and keep the numbers smaller. IT will be easier for you! y = 28
Quick review of Coordinate
Plane:
Formed by 2 perpendicular number lines that intersect at 0.
Formed by 2 perpendicular number lines that intersect at 0.
Origin: The point (0,0) where the
number lines intersect
x axis: The horizontal line
y axis: the vertical line
ordered pair: a pair of numbers that
finds a location on the coordinate plane with x first and y listed second
x coordinate: the first number in the
ordered pair that tells you how far to go the right ( or left)
y coordinate: The second number in the ordered pair that
tells you how far to go up ( or down)
Graph: Locating points on the coordinate plane to help see
the patterns (if any) between the points.
You can express the points first in a table of ordered pairs
before you graph
Here’s an example about the cost of photo CD’s where the ordered
pair (x, y) represents (number of CD’s, cost of CDs in dollars)
The sets of pairs
(1,$3), ( 2, $6) (3, $9)
Make a table
# of CDs
|
Cost in $
|
(x, y)
|
1
|
3
|
( 1, 3)
|
2
|
6
|
(2, 6)
|
3
|
9
|
(3, 9)
|
Graph the table and you will see that the points appear to
be on a straight diagonal line that slopes up from the left and to the right.
Is there a pattern?
Yes
Each point is one unit to the right and 3 units up from the
previous point. What does that mean in terms of the info about the CD’s?
It means that every time this company makes another CD it
costs them $3 more
This is called LINEAR RELATIONSHIP
This is called LINEAR RELATIONSHIP
Notice that the word linear contains the root word LINE
In order to have a graph that is a line, there must be a
constant rate of change between the change in both pieces of the data.
In the above example, the two data items are cost and number
of CD’s As the cost goes up 3, the number of CD’s goes up 1… every single time—constantly!!
So it has a constant rate of change. You make
this into a ratio where you always place the change in the y value ( the up or
down data) over the change in the x-value ( the right or left data)
So the constant rate of change for the above examples is 3/1
or $ 3/ CD
Wait—this is the unit cost!! YES IT IS!!!
Your book shows another example where Marcus downloads 2
songs each minute on his phone
The Unit Rate for this is 2 songs/ minute
If we turn this into a graph, we would graph the following points ( minutes, number of
songs)
(0 min, 0 songs) ( 1 min, 2 songs) (2 min, 4 songs) ( 3 min
6 songs) etc
The CONSTANT RATE OF
CHANGE on the graph:
As the number of songs goes UP 2 each time, the minutes go
to the RIGHT 1
Making that into a ratio up 2/ over 1 = 2/1
That’s familiar because it’s the UNIT RATE: 2 songs/ 1
minute
How can you easily tell whether it is a constant rate of
change and therefore linear? If you
graph it… it will be a diagonal line.
From the TABLE, you see the difference (change in each row)… If this is constant,
you have a linear relationship.
In the Marcus example, you would have + 2, + 2, + 2, +2 etc on the songs row and + 1, + 1, + 1, +1 etc on
the minutes row.
YOU CAN HAVE A NEGATIVE
Constant Rate of Change
Your book gives the example of taking money out of the bank
at a constant rate… or it could be a pool emptying of water at a constant rate.
Now the y value is going DOWN constantly ( or is NEGATIVE) the graph will still be a
gdiagonal, but it will look like it is going DOWN from left to right.
You can use tables and graphs to compare different ratios
Let’s say that in the previous example there is another
company that has higher costs and so it costs $4 not $3 for each CD You can produce another table:
# of CDs
|
Cost in $
|
(x, y)
|
1
|
4
|
( 1, 4)
|
2
|
8
|
(2, 8)
|
3
|
12
|
(3, 12)
|
Now graph of these
ordered pairs and compare it to the other company’s graph. Again it is a
diagonal line going up to the right But this line is STEEPER.. for every unit
to the right, it increases 4 units up… not 3 units… making it steeper WHY? Because the cost is higher..
PROPORTIONAL LINEAR RELATIONSHIPS
Linear relationships with a constant rate of change are PROPORTIONAL
if they go through the ORIGIN (0,0)
There are 2 ways to show this…on the graph see if it goes through the origin.. plug and chug to see if ( 0,0) works. or compare several points placing the y value over the x value as a ration… those ratios need o be in the same to be proportion
There are 2 ways to show this…on the graph see if it goes through the origin.. plug and chug to see if ( 0,0) works. or compare several points placing the y value over the x value as a ration… those ratios need o be in the same to be proportion
REMEMBER: The definition of a proportion is 2 equal ratios
The book gives an example which we talked about in class
Look again at page 173
Look again at page 173
Fahrenheit and Celsius temperatures
It is a LINEAR RELATIONSHIP (a diagonal line) with a
CONSTANT RATE OF CHANGE (9/5) But it is
not PROPORTIONAL because it does NOT go through the origin and if you simplify a few ratios, they are
NOT equivalent
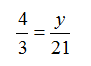
No comments:
Post a Comment