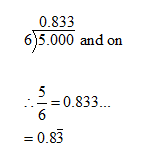Math 6 A (Periods 2 & 4)

Changing a Decimal to a Fraction 6-6

As we have seen, every fraction is equal to either a terminating decimal or a repeating decimal. It is also true that every terminating or repeating decimal is equal to a fraction.

To change a terminating decimal to a fraction in lowest terms, we write the decimal as a fraction whose denominator is a power of 10. We then write this fraction in lowest terms.

Change 0.385 to a fraction in lowest terms

0.385 = 385/1000 = 77/200

Change 3.64 to a mixed number in simple form

3.64 = 3+ 64/100 = 3 + 16/25 =  

We discovered that some fractions became repeating decimals.

For example

4/9 = 0.444444...

31/99= 0.31313131...

275/999= 0.275275275...
243/999 = 0.243243243...

and we found that you could write these with a vinculum.

so

We talked about the 9th's family.... and found the simple rule for changing a single digit decimal with the vinculum over it...

and then we saw that 

and

but we discovered that using our divisibility rules we could simplify 45/99  to 5/11

We then talked about the wonderful 11th's family and found the simple rule for changing those special fractions.

That that point we talked about

Again, using our divisibility rules we found we could simplify 243/999  because we saw that both the numerator and the denominator were divisible by 9. At that point we find that 243/999 = 27/111 But then we realize that both the numerator and the denominator are still divisible by 3. so we can simplify the fraction to 9/37.

This discussion continues with our next class! I can't wait! How about you?

Math 6A (Periods 2 & 4)

Changing a Fraction to a Decimal 6-5
There are two methods that can be used to change a fraction to a decimal.

1) find an equivalent fraction whose denominator is a power of 10. (this method does not always work but when it does it becomes really easy to change to a decimal)

13/25 multiply the numerator and the denominator by 4 to get 52/100 and then just close your eyes and see Chapter 3... and 0.52


2) divide the numerator by the denominator. It's a great way to determine your score out of 100 and then figure out your percent.

If you got 67/75 on the last test

divide 67 by 75 carefully 0.8933333... you earned a B+

take 3/8 and divide 3 by 8 8 goes into 3 0.375 times

so 3/8 = 0.375

If the numerator is smaller than the denominator we know our number must be between 0 and 1--> it must be a decimal.


When the remainder is 0 as in the case of dividing 3 by 8, it is called a terminating decimal.


By examining a fraction in lowest terms, we can determine whether the fraction can be expressed as a terminating decimal.

If the denominator has no prime factors other than 2 or 5, the decimal representation will terminate.

7/40

looking at 40 we notice the prime factorization ( oh no, it's Chapter 5)
40 = 2³·5 Since the only prime factors are 2 and 5

7/40 must terminate.

What about 5/12 ?

12 = 2²· 3 Since 3 is a prime factor of the denominator, the fraction cannot be expressed as a terminating decimal.


What about 9/12 ? At first it looks the same as the one above, but look carefully and realize 9/ 12 = 3/4

Since 4 = 2² , 4 has no other prime factors except 2, this can be expressed as a terminating decimal.


Let's look at 15/22
Since 22 has the prime factor of 11 we know that this will not terminate. In fact when you divide 15 by 22 you end up with 0.681818181...


We write this as ( Please check page 196) Notice that the bar is only over the 81 and represents a block  numbers that continues to repeat indefinitely and is called a repeating decimal.


EVERY FRACTION CAN BE EXPRESSED AS EITHER A TERMINATING DECIMAL OR A REPEATING DECIMAL.


Let's look at 4/9 = 0.4444444....

5/9 =

7/9 =


31/99 = 0.3131313131...

8/ 11 = 72/99 = .72727272...

Algebra Honors (Periods 5 & 6)

Decimal Forms of Rational Numbers 11-2

Any common fraction can be written as a decimal by dividing the numerator by the denominator. If the remainder is zero, the decimal is called a terminating, or ending, or finite decimal.

3/8=  0.375

Actually this is one of the fractions you need to know by heart !

If you don’t reach a remainder of zero when dividing the numerator by the denominator, continue to divide until the remainder begins to repeat.

5/6

  

7/11

The decimal quotient above are nonterminating,  nonending, or infinite. The dots indicate that the decimals continue without end.

They are also called repeating or periodic because the same digit or block of digits repeats unendingly. A bar (vinculum) is used to indicate the block of digits that repeat.

What ones do you need to know by heart… same from 6^th grade

1/3 family,   1/11 family,    and    let’s look at the 1/7 family (my favorite)

Let’s look at this algebraically… when you divide a positive integer n by a positive integer d, the remainder  r   at each step must be zero or a positive integer less than d.  For example, if the divisor is 6, the reminders will be 0, 1, 2, 3, 4, or 5 and the division will terminate or begin repeating within 5 steps after only zeros remain to be brought down.  Think about this!

For every integer   n and every positive integer   d, the decimal form of the rational number n/d either terminates or eventually repeats in a block of fewer than d digits.

To express a terminating decimal as a common fraction, express the decimal as a common fraction with a power of ten as the denominator. Then express in simplest form

                                                         

To express a repeating decimal follow these steps :  

Try it with  

You should get    179/330

How about     ? 

This one can be done easily if you remember the rule of matching the block of repeating digits with the digits in the decimal.

In this case        

All terminating and all repeating decimals represent rational numbers that can be written in the form n/d where n is an integer and d is a positive integer.

It is often convenient or even required that you use an approximation of a lengthy decimal. For example, you may approximate 7/13 as 0.53846, or 0.538, or 0.54       

As review: to round a decimal

1.       if the first digit dropped is greater than or equal to 5, add a 1 to the last digit retained.

2.       if the first digit dropped is less than 5, don’t change the last digit retained

Use the symbol    ≈   which means    “is approximately equal to”

Math 6A (Periods 2 & 4)

Comparing Fractions 6-4
When two fractions have equal denominators it is easy to tell which of the fractions is greater.
We simply compare their numerators.
3/11 < 5/11 since 3< 5 If the fractions have different denominators, there are a variety of methods to consider. We could find a common denominator, which we will need to do when we add or subtract fractions... but when comparing let's try other methods... Take 2/3 and 4/5 Comparing Fractions


Or compare 5/6 and 7/9

again this time you would multiply
5(9) = 45 and 7(6) = 42
so 5/6 > 7/9

Also if the numerator is the same
2/3, 2/7, 2/9, 2/11, 2/21, 2/35

The larger the denominator the smaller the fractions so to list in order from least to greatest start with the largest number in the denominator!!

and if you have fractions with the numerator just one away from the denominator
such as 3/4, 5/6, 7/8, 9/10, 23/24, 45/46
the smallest fraction will be the one with the smallest numbers
3/4 is the smallest fraction and that list is in order from least to greatest!!

What if you need to name a fraction between two fraction 1/6 and 3/8
you could find the LCD
1/6 = 4/24 and
3/8 = 9/24
so you could state
5/24, 6/24 ( but that is really 1/4), 7/24, or 8/24 ( but that is really 1/3.
There are actually an infinite number of fractions... these are only 4 of them

What if you need to find a fraction between 3/7 and 4/7
sometimes you need to change the denominators just to realize that there really are other fractions between
for instance, 3/7 = 6/14 and 4/7 = 8/ 14 so doesn't 7/14 ( or actually 1/2) work!!
... and that's just one of the fractions!!

If n > 0
Then
if a < b a/n < b/n Think about this one!! Plug in some numbers and see what happens and if a < b, then n/a > n/b
Again, plug in some numbers and see what happens!!

If a/b and c/d are fractions and if ad > bc, which fraction is greater,
a/b or c/d ?
Post your answer below in the comments for extra credit. Make sure to give your reasoning for your answer.


Ordering or comparing fractions:

Different ways:

I. Benchmarks - 0, 1/4, 1/2, 3/4, and 1 (using your gut feeling)
How do you figure out which benchmark to use?

When the numerator is close to the denominator, the fraction is approaching 1

(Ex: 9/11)

When you double the numerator and it's close to the denominator, the fraction is close to 1/2
(Ex: 4/9)

When the numerator is very far from the denominator, the fraction is approaching zero
(Ex: 1/8)

Also, if one number is improper or mixed number and other is a proper fraction,
then obviously the number greater than 1 will be bigger!

II. LCD - give them all the same denominator using the LCM as the LCD


III. Use cross multiplication when comparing two... do it several times when comparing a list of fractions

IV. Change them to decimals ( works well if you are great at decimals-- but I want you to become GREAT at fractions!!)

Algebra Honors (Periods 5 & 6)

Properties of Rational Numbers 11-1

A real number that can be expressed as the quotient of two integers is called a rational number

A rational number can be written as a quotient of integers in an unlimited number of ways.

To determine which of two rational numbers is greater, you can write them with the same positive denominator and compare the numerators

Which is greater                                                           

?

the LCD is 21   

  

  

For all  integers  a and b and all positive integers c and d

   if an only if ad > bc

 if and only if ad < bc

This method compares the product of the extremes with the product of the means

Thus 4/7 > 3/8 because (4)(8) > (3)(7)

Rational Numbers differ from Integers in several ways. For example, given an integer, there IS a next greater integer.

That is, -8 is greater than -9. 1 follows 0, 35 follows 34 and so on. There is no “Next Greater” rational number after a given rational number.

The Density Property for Rational Numbers
Between every pair of different rational numbers there is another rational number

The density property implies that it is possible to find an unlimited or endless number of rational numbers between two given rational numbers.

If a and b are rational numbers and a < b  then the number halfway from a to b is

 and the number one third of the way from a to b would be

and so on. 

Math 6High ( Period 3)

Comparing Positive and Negative Numbers 4.8

Up until now we have focused on positive and negative Integers—now we will look at Rational Numbers – negative Fractions, negative Decimals and negative Mixed Numbers!

Please review Page 202 for graphing Negative Decimals and Negative Fractions

Comparing Positive and Negative Numbers

Complete the statements using < , >,  or =

-1/6  ? -0.6

-9/8  ?  -1.125

-2 1/3  ?  -2.1

To find the correct solution for each of the above, rewrite the fractions and mixed numbers as decimals. Then graph the numbers on a number line and compare.

-1/6 is    - 0.1666… or rounded   -0.17

-0.17 is to the right of -0.6 so

-1/6 > -0.6

-9/8 = -1 1/8 = -1.125

so -9/8 is at the same point on the number line as -1.125 so

-9/8 = -1.125

-2 1/3 = -2.33333 or rounded to -2.3 which is to the left of -2.1

so

-2 1/3 < -2.1

Ordering Positive and Negative Numbers

Write  1.3 ,     -2 ½ ,  - 2.6  and 3/8 in order from least to greatest.

Solution

1.  First rewrite the fractions as decimals ( or the decimals  as fractions)

-2½ = -2.5  and 3/8 = 0.375

2. Then draw a number line. You can estimate to plot the points.

(See our textbook page 203)

From least to greatest

-2.6,    -2 ½ ,   3/8,   1.3

Algebra Honors (Periods 5 & 6)

Complex Fractions Chapter 6 Extra 

A complex fraction is a fraction whose numerator or denominator  contains one or more fractions. To express a complex fraction as a simple faction use one of the following methods:

Method 1:  Simply the numerator and the denominator.  Express the fraction as a quotient using the division symbol  ÷ .   Multiply by the reciprocal of the divisor. 

Method 2: Find the LCD of ALL the simple fractions in the complex    fraction. Multiply the numerator and the denominator of the complex  fraction by the LCD 

Using Method 1

Method 1:  Simply the numerator and the denominator.  Express the fraction as a quotient using the division symbol  ÷ .   Multiply by the reciprocal of the divisor. 

Simplify above to get

Using Method 2

Method 2: Find the LCD of ALL the simple fractions in the complex    fraction. Multiply the numerator and the denominator of the complex  fraction by the LCD 

   

Same problem as above but this time Find the LCD of all the simple fractions in the complex fraction... The LCD would be 2ab. Multiply each simple fraction by 2ab

Which simplifies to

Then you can factor:

Hopefully you realize you have been able to obtain the same simplified fraction using EITHER method. Use whichever method works BEST for you!